EM 1110-2-1100 (Part II)
30 Apr 02
primarily on two factors. One factor is the ratio of the volume of water that enters on one tidal cycle (the tidal
prism) to the total volume of water in the harbor. This ratio, in turn, will depend on the tide range, the
hydraulic efficiency of the harbor entrance, and the water depths in the harbor. Generally, the larger the value
of this ratio, the more exchange that will occur. The other factor is the momentum of the incoming jet of
water on the rising tide, and the consequent amount of penetration of this jet and its resulting angular
momentum as it establishes a rotating gyre inside the harbor. The strength of this jet is related to the amount
of flushing that will occur and the strength of the tidally induced circulation in the harbor.
(b) The most commonly used factor for defining the effectiveness of tidally induced harbor flushing is
the average per cycle exchange coefficient E (Nece and Richey 1975) given by
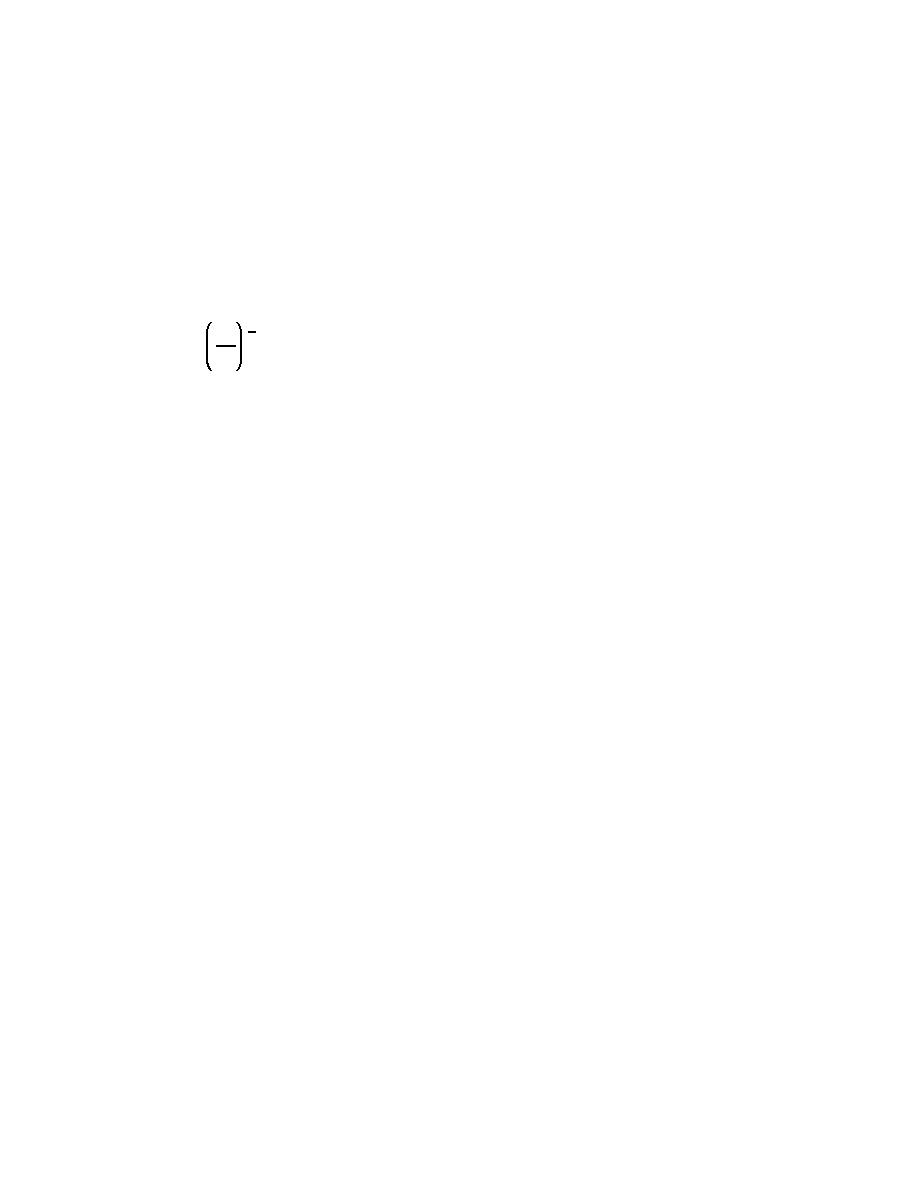
1
Ci
i
E'1&
(II-7-20)
Co
where Co is the initial concentration of some substance in the harbor water and Ci is the concentration of this
substance after i tidal cycles. E may be defined at a point in the harbor by using concentration values at that
point or may be defined for the entire harbor by using spatially average concentration values for the entire
harbor. E is the fraction of harbor water removed each tidal cycle. Equation II-7-20 assumes essentially
repetitive identical tides and no further addition of the marker substance to the harbor.
(c) As an example of the use of the exchange coefficient, consider Figure II-7-39, which is modified from
Falconer (1980) and based on physical and numerical model results. Figure II-7-39 shows the spatial average
exchange coefficient for a rectangular harbor having the dimensions L and B shown in the insert. Note where
the harbor entrance is located. E has a peak value of about 0.5 when L/B is near unity (i.e. a square harbor).
For L/B values outside the range of 0.3 to 3, the flushing of the harbor is much less effective. The tidal prism
ratio (TPR), defined as the tidal prism divided by the total harbor volume at high tide, was 0.4 for the results
shown in Figure II-7-39.
(d) Another factor used to define the amount of flushing is the "flushing efficiency," defined as the
exchange coefficient divided by the tidal prism ratio (i.e. E/TPR) expressed as a percentage (Nece, Falconer,
and Tsutumi 1976). Flushing efficiency compares the actual water exchange in one cycle with the volume
of exchange that would occur if the incoming tidal prism completely mixed with the harbor water on each
cycle. Table II-7-3 shows some results for five small-boat harbors in the state of Washington. These results
are based on model tests (Nece, Smith, and Richey 1980).
(e) Note that the exchange coefficients are all around 0.2, which is below the value for the more idealized
rectangular harbors (Figure II-7-39). And, for relatively consistent exchange coefficients, the flushing
efficiency showed a much greater range of values. For the rectangular harbor in Figure II-7-39 where the
TPR is 0.4, the flushing efficiency would vary from 0 up to about 125 percent. The desirable exchange
coefficient or flushing efficiency for a given harbor would depend on the level of pollution in the harbor
versus the desired harbor water quality. Although higher exchange coefficients and flushing efficiencies
generally indicate better harbor flushing by tidal action, there may still be small pockets of stagnant water
in a generally well-flushed harbor.
(2) Wind effects.
(a) Wind acting on the water surface will generate a surface current that, in water depths typically found
in harbors, will essentially be in the direction of the wind. Owing to Coriolis effects, the current will be a few
degrees to the right of the wind in the Northern Hemisphere; e.g., see Neumann and Pierson (1966) or
Bowden (1983). If the distance over which the wind blows and the wind duration are sufficient, the surface
Harbor Hydrodynamics
II-7-49


 Previous Page
Previous Page
