Wave height distribution.

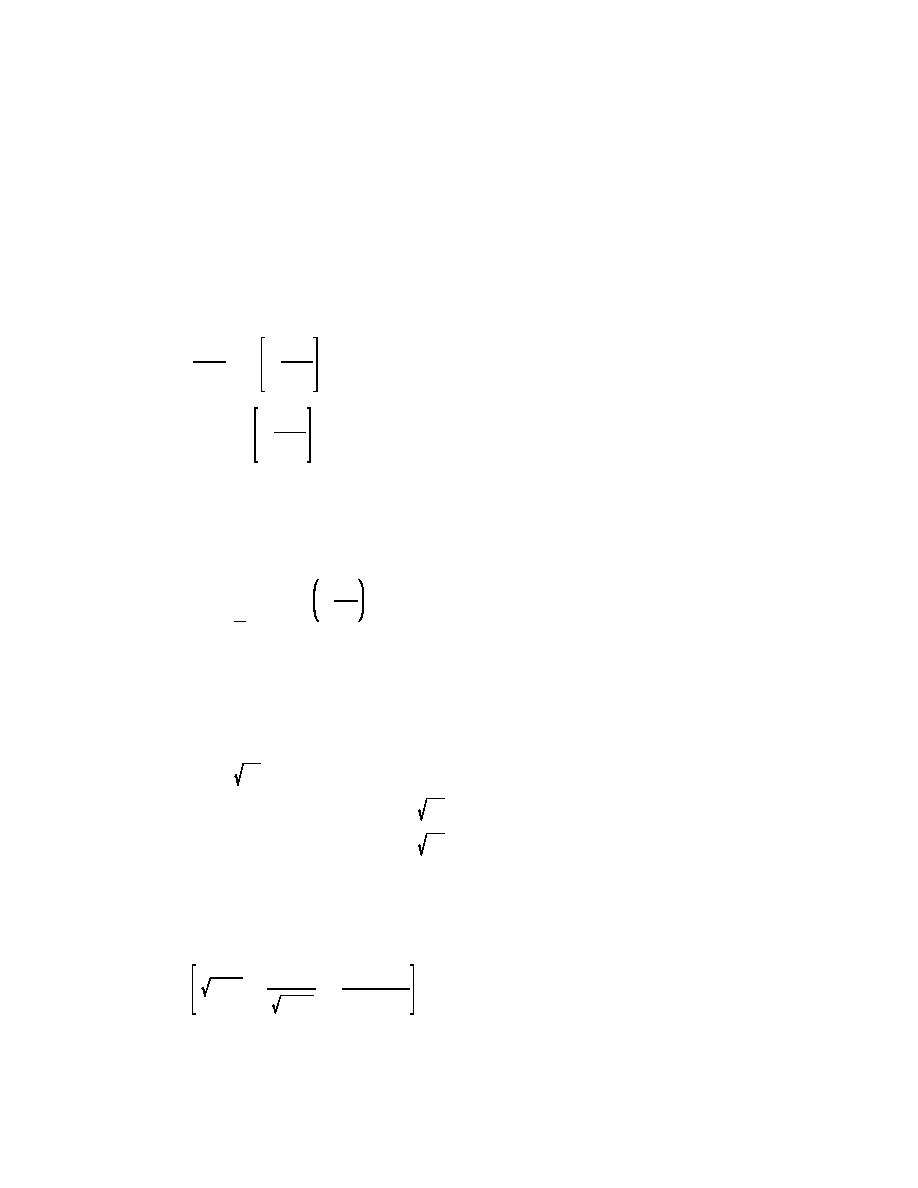
EM 1110-2-1100 (Part II)

30 Apr 02

(7) Wave height distribution.

(a) The heights of individual waves may be regarded as a stochastic variable represented by a

probability distribution function. From an observed wave record, such a function can be obtained from a

histogram of wave heights normalized with the mean heights in several wave records measured at a point

(Figure II-1-30). Thompson (1977) indicated how well coastal wave records follow the Rayleigh distribution.

If wave energy is concentrated in a very narrow range of wave period, the maxima of the wave profile will

coincide with the wave crests and the minima with the troughs. This is termed a narrow-band condition.

Under the narrow-band condition, wave heights are represented by the following Rayleigh distribution

(Longuet-Higgins 1952, 1975b, 1983)

H2

2H

exp &

p(H) '

2

Hrms

(II-1-130)

H2

P(H) ' 1 & exp &

2

Hrms

(b) The significant wave height H1/3 is the centroid of the area for H $ H* under the density function

where H > H* corresponds to waves in the highest one-third range as shown in Figure II-1-29, that is

2

H(

&

1

2

Hrms

P(H() ' 1 &

(II-1-131)

'1&e

3

from which we find H* = 1.05Hrms. Various estimates of wave heights may then be obtained upon integration

of the above equation using certain mathematical properties of the Error function (Abramowitz and Stegun

1965). We find

H1/3 . 4.00 m0 ' 1.416 Hrms

H1/10 ' 1.27 H1/3 ' 1.80 Hrms ' 5.091 m0

(II-1-132)

H1/100 ' 1.67 H1/3 ' 2.36 Hrms ' 6.672 m0

Hmax ' 1.86 H1/3

(for 1000 wave cycles in the record)

(c) The most probable maximum wave height in a record containing N waves is related to the rms wave

height (Longuet-Higgins 1952) by

0.2886

0.247

logN %

Hrms

Hmax '

(II-1-133)

&

(logN)3/2

logN

(d) The value of Hmax obtained in this manner can be projected to a longer period of time by adjusting

the value of N based on the mean zero-upcrossing period (Tucker 1963).

II-1-74

Water Wave Mechanics