EM 1110-2-1100 (Part II)
(Change 1) 31 July 2003
where
Ugr = gradient approximation to the wind speed
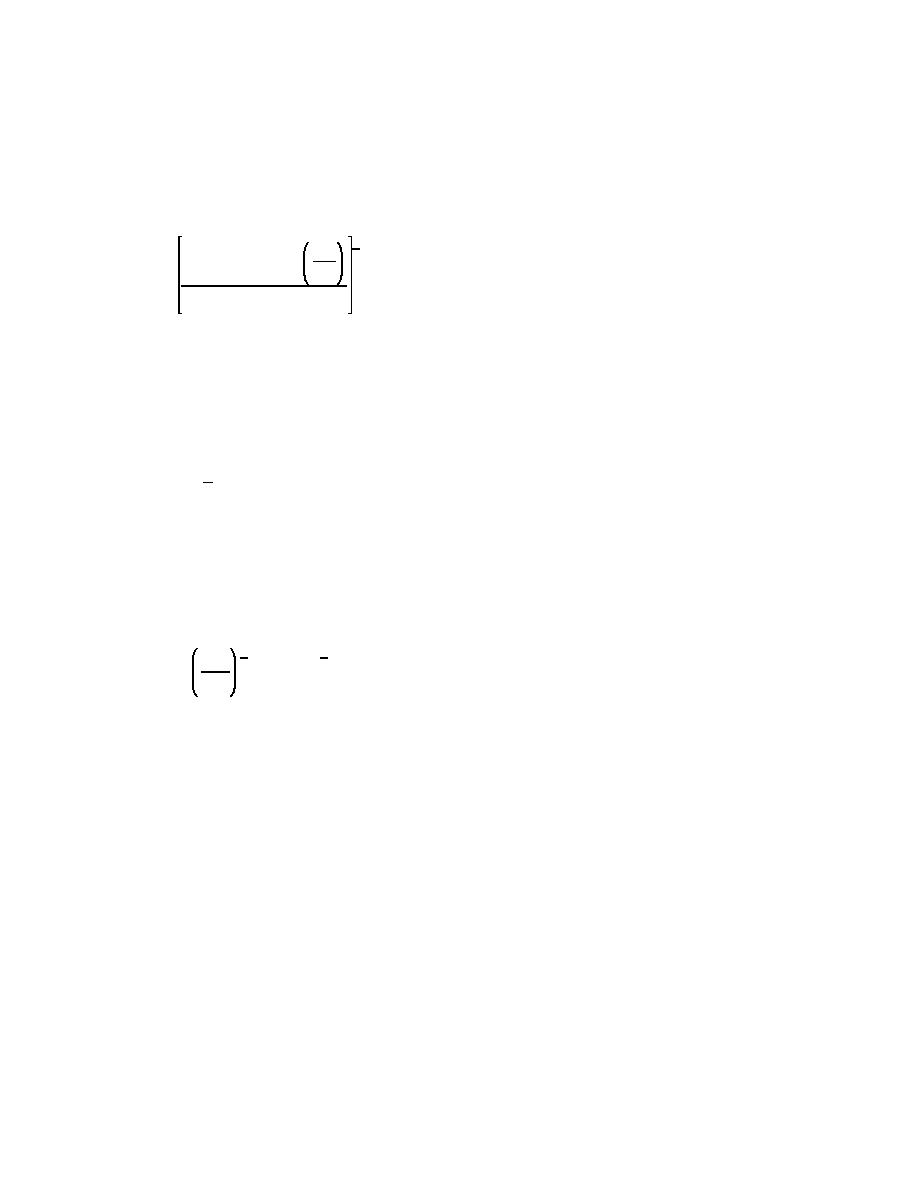
(7) In the intense portion of the storm, Equation II-2-18 reduces to a cyclostrophic approximation given
by
1
&A
2
A B (pn & pc) exp
rB
Uc '
(II-2-19)
ρa r B
where
Uc = cyclostrophic approximation to the wind speed
which yields explicit forms for the radius to maximum winds as
1
B
Rmax ' A
(II-2-20)
where
Rmax = distance from the center of the storm circulation to the location of maximum wind speed
(8) The maximum wind speed can then be approximated as
1
1
B
2
2
(pn & pc)
Umax '
(II-2-21)
ρa e
where
Umax = maximum velocity in the storm
e = base of natural logarithms, 2.718
(9) Rosendal and Shaw (1982) showed that pressure profiles and wind estimates from the Holland
model appeared to fit observed typhoon characteristics in the central North Pacific. If B is equal to 1 in this
model, the pressure profile and wind characteristics become similar to results of Myers (1954); Collins and
Viehmann (1971); Schwerdt, Ho, and Watkins (1979); and Cardone, Greenwood, and Greenwood (1992).
In the case of the Cardone, Greenwood, and Greenwood model, this similarity would exist only for the case
of a storm with no significant background pressure gradient.
(10) Holland argues that B=1 is actually the lower limit for B and that, in most storms, the value is likely
to be more in the range of 1.5 to 2.5. As shown in Figure II-2-16, this argument is supported by the data from
Atkinson and Holliday (1977) and Dvorak (1975) taken from studies of Pacific typhoons. The effect of a
higher value of B is to produce a more peaked wind distribution in the Holland model than exists in models
with B set to a value of 1. According to Holland (1980), use of a wind field model with B=1 will
underestimate winds in many tropical storms. In applications, the choices of A and B can either be based on
Meteorology and Wave Climate
II-2-29


 Previous Page
Previous Page
