EM 1110-2-1100 (Part II)
30 Apr 02
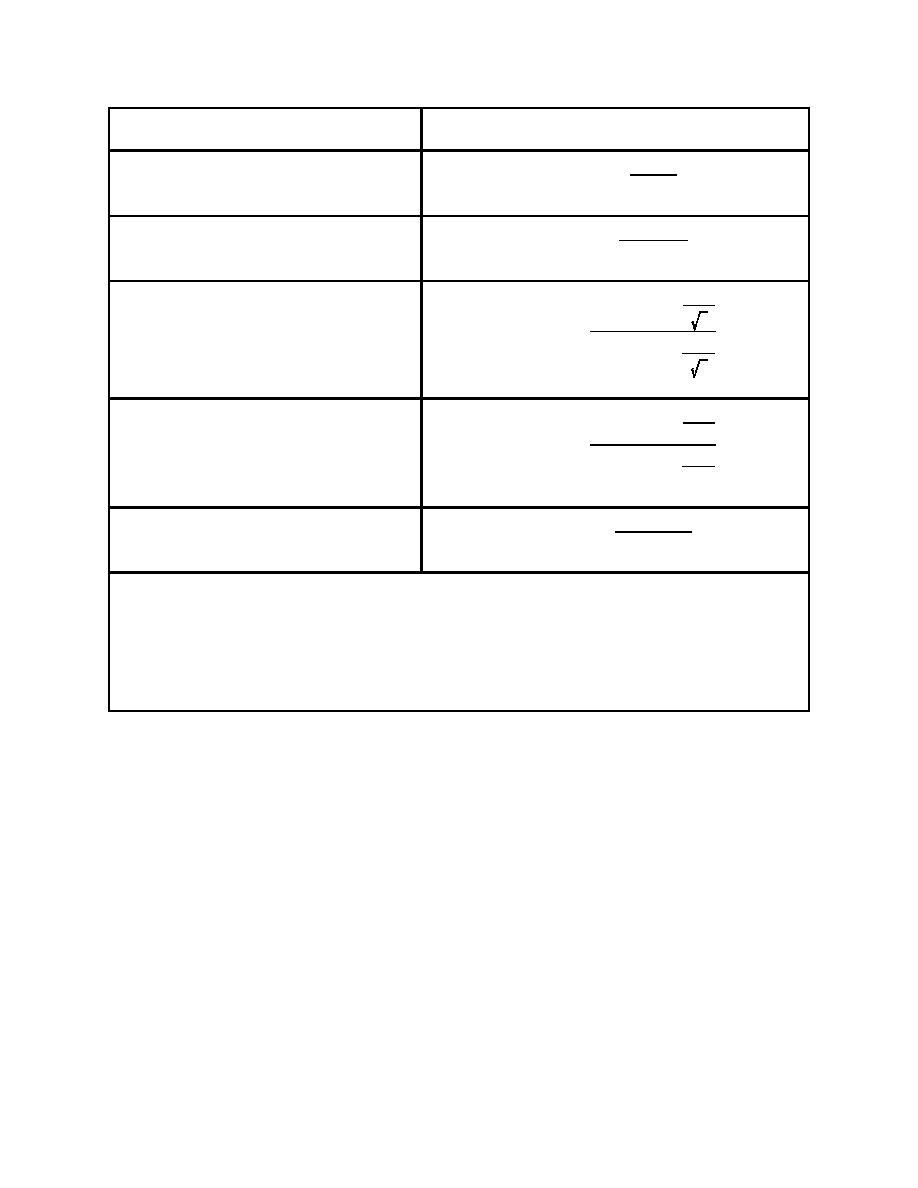
Application of Formula
Plotting Position Formula
m
Traditional
^
Fm ' 1 &
(Gumbel 1958)
N%1
m & 0.44
Fisher-Tippett I (FT-I)
^
Fm ' 1 &
(Gringorten 1963)
N % 0.12
0.27
m & 0.20 &
k
^
Fm ' 1 &
Weibull Distribution Function
0.23
N % 0.20 %
(Goda 1988)
k
0.52
m & 0.11 &
Fisher Tippett II (FT-II)
k
^
Fm ' 1 &
or Frechet Distribution Function
0.11
N % 0.12 &
(Goda and Kobune 1990)
k
m & 0.375
Log-Normal Distribution Function
^
Fm ' 1 &
(Blom 1958)
N % 0.25
Parameter definitions:
^
m = rank of data value in descending order (m = 1 for largest, etc.)
N = number of events1
k = parameter in Weibull distribution function
1
For censored data, N should represent the total number of events over the time interval considered
(not just the number of censored events)
Figure II-8-5. Plotting position formulas
prudent to plot the computed distribution function and data together and ensure that the fit is consistent with
good engineering judgement.
(4) Outliers. Outliers are retained in the data, but they should receive special scrutiny, as follows:
(a) Ensure accuracy. Each outlier should be checked to ensure that it is a valid data value, rather than
a measurement or modeling error.
(b) Examine each event that produces a high outlier. Typical causes are very severe winter storms or
direct impact of an intense hurricane. If extreme events at the site are produced by distinctly different natural
processes (different statistical populations), it may be preferable to divide the data values into several series,
one for each process, and analyze each series separately (e.g. Goda 1988). For example, winter storms and
hurricanes should not necessarily be expected to produce extremes that follow the same extremal probability
distribution function. Extreme data values can be analyzed as separate populations only if sufficient data
values are available in each population.
Hydrodynamic Analysis and Design Conditions
II-8-11


 Previous Page
Previous Page
