EM 1110-2-1100 (Part II)
30 Apr 02
b. Definition of wave parameters.
(1) A progressive wave may be represented by the variables x (spatial) and t (temporal) or by their
combination (phase), defined as θ = kx - ωt, where k and ω are described in the following paragraphs. The
values of θ vary between 0 and 2π. Since the θ-representation is a simple and compact notation, it will be
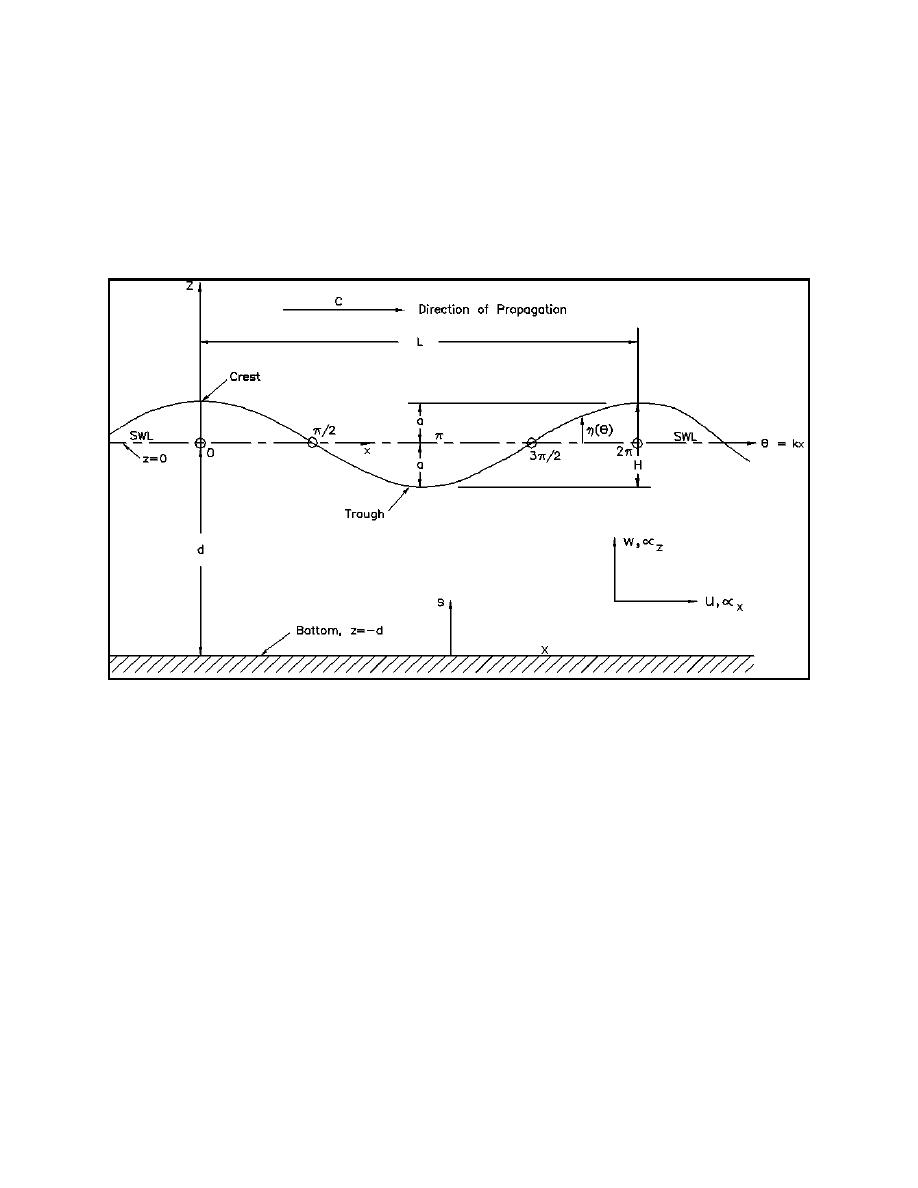
used in this chapter. Figure II-1-1 depicts parameters that define a simple, progressive wave as it passes a
fixed point in the ocean. A simple, periodic wave of permanent form propagating over a horizontal bottom
may be completely characterized by the wave height H wavelength L and water depth d.
Figure II-1-1.
Definition of terms - elementary, sinusoidal, progressive wave
(2) As shown in Figure II-1-1, the highest point of the wave is the crest and the lowest point is the
trough. For linear or small-amplitude waves, the height of the crest above the still-water level (SWL) and
the distance of the trough below the SWL are each equal to the wave amplitude a. Therefore a = H/2, where
H = the wave height. The time interval between the passage of two successive wave crests or troughs at a
given point is the wave period T. The wavelength L is the horizontal distance between two identical points
on two successive wave crests or two successive wave troughs.
(3) Other wave parameters include ω = 2π/T the angular or radian frequency, the wave number k = 2π/L,
the phase velocity or wave celerity C = L/T = ω/k, the wave steepness ε = H/L, the relative depth d/L, and the
relative wave height H/d. These are the most common parameters encountered in coastal practice. Wave
motion can be defined in terms of dimensionless parameters H/L, H/d, and d/L; these are often used in
practice. The dimensionless parameters ka and kd, preferred in research works, can be substituted for H/L
and d/L, respectively, since these differ only by a constant factor 2π from those preferred by engineers.
II-1-4
Water Wave Mechanics


 Previous Page
Previous Page
