EM 1110-2-1100 (Part II)
30 Apr 02
Table II-1-1
Classification of Water Waves
Classification
d/L
kd
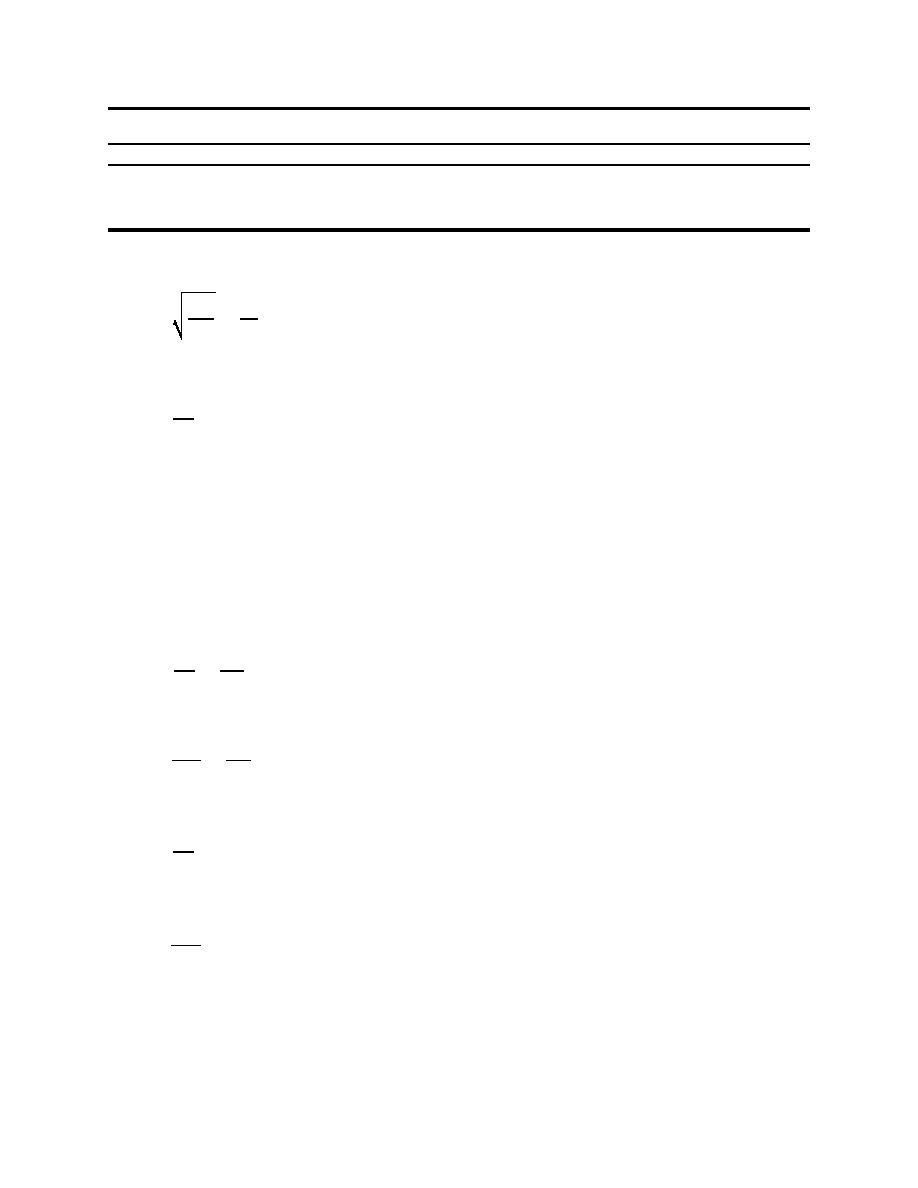
tanh (kd)
1/2 to 4
π to 4
.1
Deep water
Transitional
1/20 to 1/2
π/10 to π
tanh (kd)
. kd
Shallow water
0 to 1/20
0 to π/10
(i) In deep water, tanh (kd) approaches unity, Equations II-1-7 and II-1-8 reduce to
gL0
L0
C0 '
(II-1-12)
'
2π
T
and Equation II-1-9 becomes
gT
C0 '
(II-1-13)
2π
(j) Although deep water actually occurs at an infinite depth, tanh (kd), for most practical purposes,
approaches unity at a much smaller d/L. For a relative depth of one-half (i.e., when the depth is one-half the
wavelength), tanh (2πd/L) = 0.9964.
(k) When the relative depth d/L is greater than one-half, the wave characteristics are virtually
independent of depth. Deepwater conditions are indicated by the subscript 0 as in Lo and Co except that the
period T remains constant and independent of depth for oscillatory waves, and therefore, the subscript for
wave period is omitted (Ippen 1966). In the SI system (System International or metric system of units) where
units of meters and seconds are used, the constant g/2π is equal to 1.56 m/s2 , and
gT
9.8
C0 '
(II-1-14)
T ' 1.56 T m/s
'
2π
2π
and
gT 2
9.8 2
T ' 1.56 T 2 m
L0 '
(II-1-15)
'
2π
2π
(l) If units of feet and seconds are specified, the constant g/2π is equal to 5.12 ft/s2 , and
gT
C0 '
(II-1-16)
' 5.12 T ft/s
2π
and
gT 2
' 5.12 T 2 ft
L0 '
(II-1-17)
2π
(m) If Equations II-1-14 and II-1-15 are used to compute wave celerity when the relative depth is d/L =
0.25, the resulting error will be about 9 percent. It is evident that a relative depth of 0.5 is a satisfactory
boundary separating deepwater waves from waves in water of transitional depth. If a wave is traveling in
transitional depths, Equations II-1-8 and II-1-9 must be used without simplification. As a rule of thumb,
Equation II-1-8 and II-1-9 must be used when the relative depth is between 0.5 and 0.04.
II-1-8
Water Wave Mechanics


 Previous Page
Previous Page
