Fourier approximation -- Fenton's theory. (cont)

EM 1110-2-1100 (Part II)

30 Apr 02

consistently and better than results from other theories for a wide range of wave height, wave period, and

water depth. Based on these comparisons, Fenton and McKee (1990) define the regions of validity of Stokes

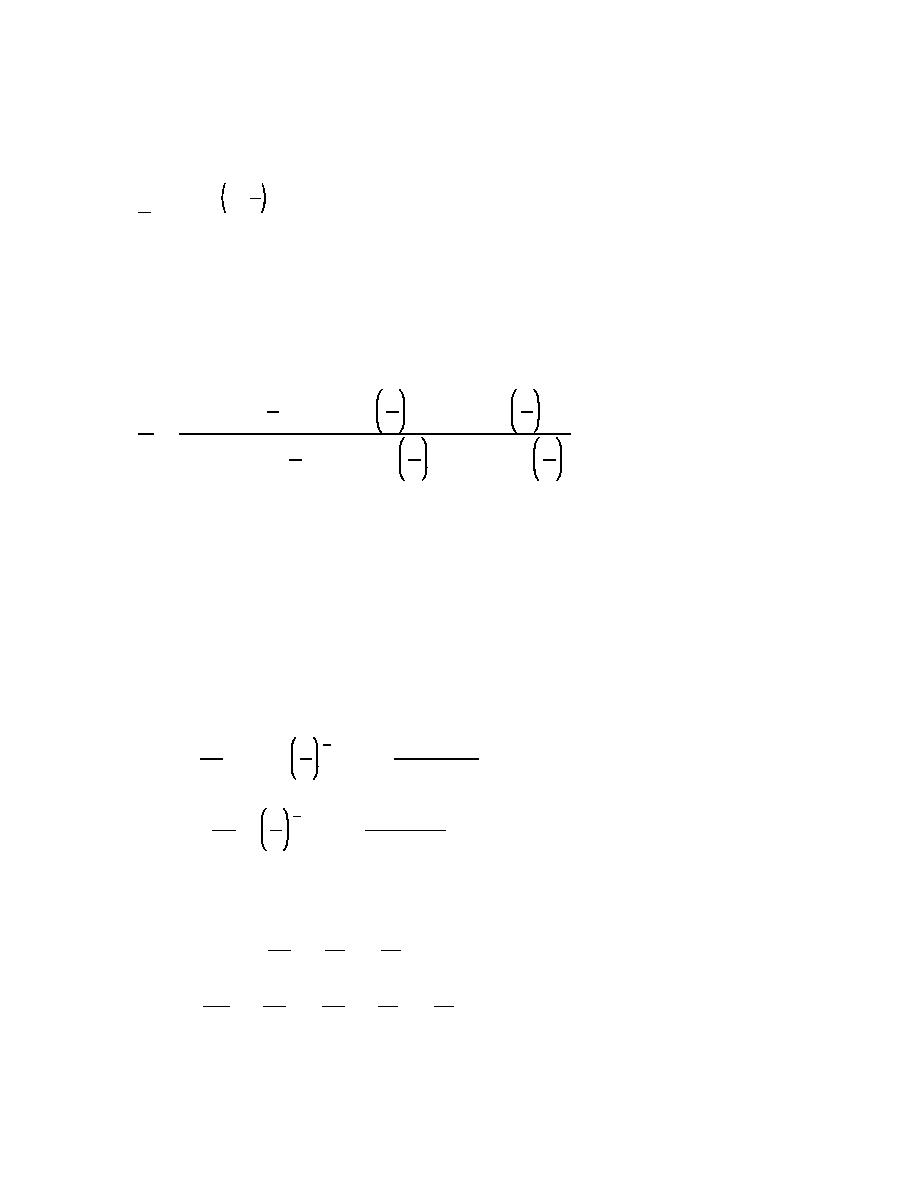
and cnoidal wave theory as

H

&1.87

L

d

' 21.5 e

(II-1-104)

d

(n) The cnoidal theory should be used for wavelengths longer than those defined in this equation. For

shorter waves, Stokes' theory is applicable. Fenton's theory can be used over the entire range, including

obtaining realistic solutions for waves near breaking.

(o) In water of finite depth, the greatest (unbroken) wave that could prevail as a function of both

wavelength and depth is determined by Fenton and McKee (1990) as

2

3

L

0.141063 % 0.0095721

% 0.0077829

H

d

a

(II-1-105)

'

d

2

3

L

1.0 % 0.078834

% 0.0093407

% 0.0317567

d

(p) The leading term in the numerator of this equation is the familiar steepness limit for short waves in

deep water. For large values of L/d (i.e., shallow-water waves), the ratio of cubic terms in the above equation

approaches the familiar 0.8 value, a limit for depth-induced breaking of the solitary waves. Therefore, the

above equation may also be used as a guide to delineate unrealistic waves in a given water depth.

(q) The formulas for wave kinematics, dynamics, and wave integral properties for Fenton's theory have

been derived and summarized (Sobey et al.1987; Klopman 1990). Only the engineering quantities of interest

including water particle velocities, accelerations, pressure, and water surface elevation defined relative to a

Eulerian reference frame are provided here.

(r)

The horizontal and vertical components of the fluid particle velocity are

1

j jBj

N

MΨ

g

cosh jk(z%d)

2

' &u %

cos jkx

u(x,z) '

(II-1-106)

Mz

k

cosh jkd

j'1

1

j jBj

N

MΨ

g

sinh jk(z%d)

2

w(x,z) ' &

(II-1-107)

sin jkx

'

Mx

k

cosh jkd

j'1

(s) Fluid particle accelerations in the horizontal and vertical directions are found by differentiating the

velocities and using the continuity equation. These component accelerations are

Mu

Du

'u

%w

ax (x,z) '

Mx

Mz

Dt

(II-1-108)

Mw

Mu

Dw

'u

%w

'u

&w

az(x,z) '

Mx

Mz

Mx

Dt

where

Water Wave Mechanics

II-1-53