EM 1110-2-1100 (Part II)
30 Apr 02
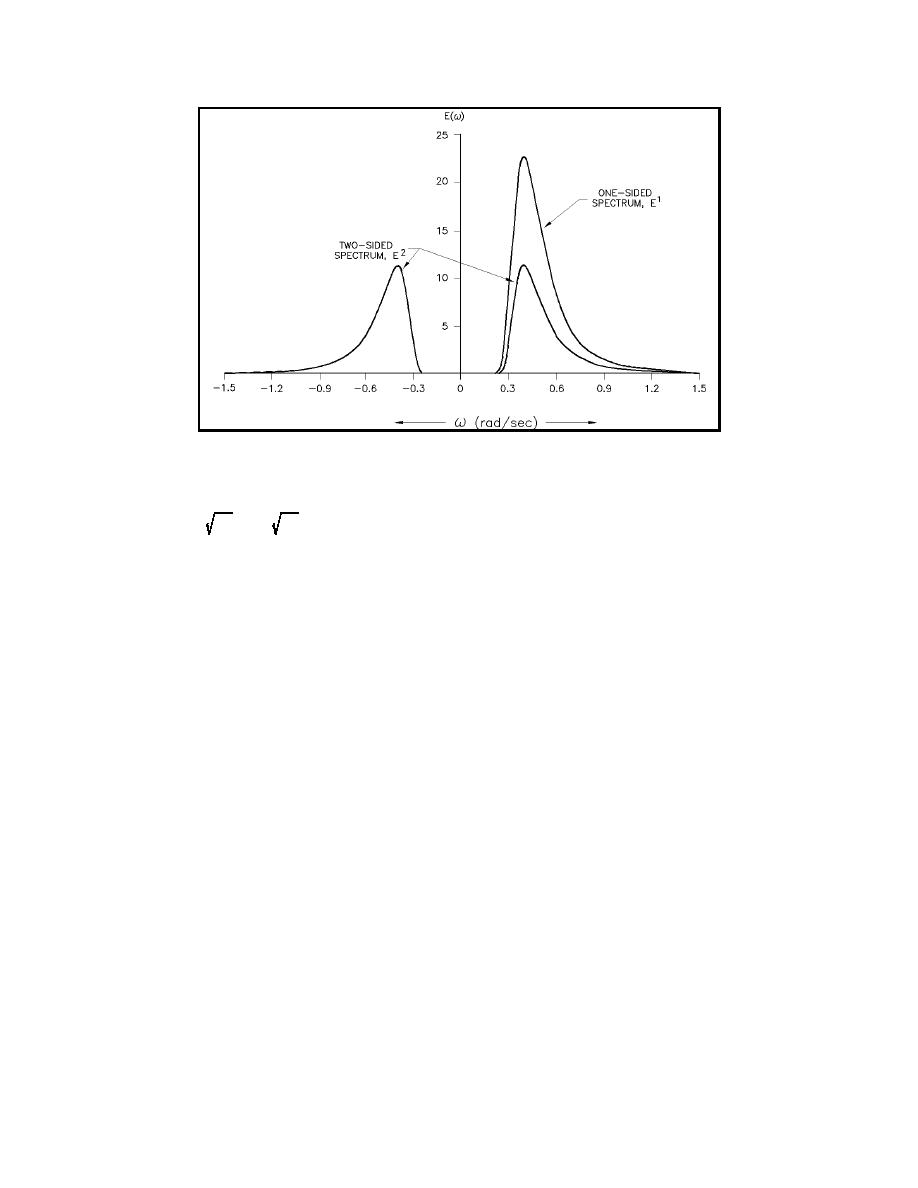
Figure II-1-36. Definition of one- and two-side wave spectrum (Chakrabarti 1987)
Hs ' 3.8 m0 . 4 m0
(II-1-149)
(n) Therefore, the zero-th moment m0, which is the total area under the wave energy density spectrum,
defines the significant wave height for a given E(f) (Figure II-1-37).
(3) Examples of frequency spectra. The frequency spectrum is normally plotted as energy density on
the ordinate versus frequency on the abscissa (Figures II-1-31 through II-1-37). In principal, the form of E(f)
can be quite variable. However, some generalizations are possible. First of all, during strong wind events,
the spectrum tends to have a strong central peak and a fairly predictable shape. For swell that has propagated
a long distance from the source of generation, waves tend to have a single sharp peak. Waves in shallow
water near breaking tend to have a sharp peak at the peak frequency fp and have a series of smaller peaks at
frequencies 2 fp, 3 fp, etc., which are harmonics of the main wave. The presence of harmonics indicates that
the wave has the sharp crest and flat trough of highly nonsinusoidal waves often found near breaking. To
complicate matters, Thompson (1977) has shown that about two-thirds of U.S. coastal wave records have
more than one peak, indicating the presence of multiple wave trains. These wave trains most likely originated
from different areas and have different directions of propagation. Moreover, it is possible to have a single-
peak spectrum, which consists of two trains of waves of about the same frequency but different directions of
propagation. In order to sort these issues out, observations of the directional spectrum are required. Figures
II-1-31, II-1-32, and II-1-35 include examples of different frequency spectra providing some indication of
their range of variability.
(4) Wave spectrum and its parameters.
(a) Two parameters are frequently used in the probability distribution for waves. These are the spectral
width ν and the spectral bandwidth ε, and are used to determine the narrowness of a wave spectra. These
parameters range from 0 to 1, and may be approximated in terms of spectral moments by
Water Wave Mechanics
II-1-85


 Previous Page
Previous Page
