EM 1110-2-1100 (Part II)
30 Apr 02
(Hudspeth and Chen 1979) are two alternatives for simulating random waves from a given one-dimensional
spectrum.
(c) There are two ways for simulating wave surface profiles from known wave spectra; deterministic
and non-deterministic spectral amplitude methods. In the deterministic spectral simulation method, the wave
height, period, and phase angle associated with a frequency f1 whose corresponding energy density is E(f1)
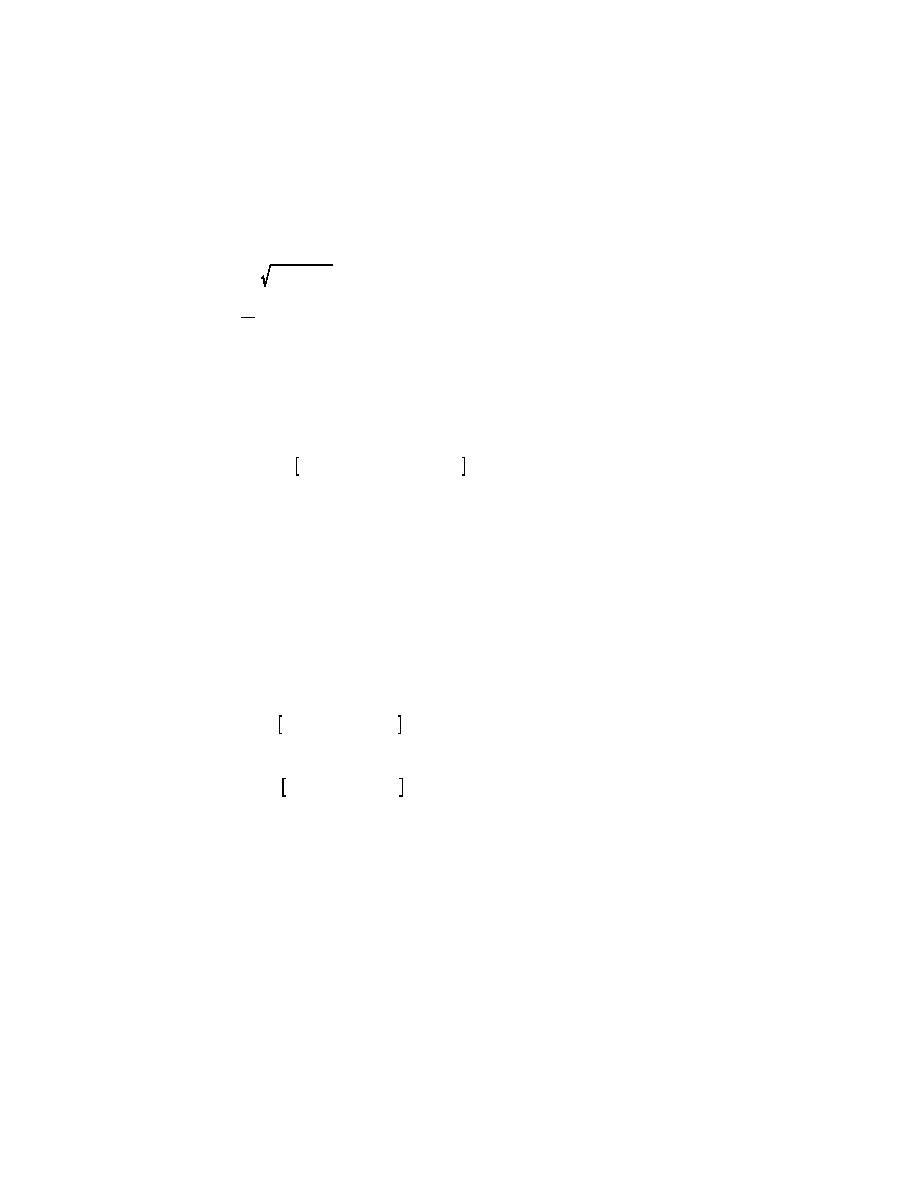
may be obtained from
H( f1) ' H*f ' 2 2E(f1) ∆f
1
1
(II-1-174)
T (f1) ' T*f '
f1
1
ε (f1) ' ε*f ' 2πrN
1
where the phase angle ε is arbitrary since rN is a random number between zero and one. The time series of
the wave profile at a point x and time t may be computed by (Tucker et al. 1984)
η (x,t) ' j H(n) cos k (n) x & 2π f (n) t % ε(n)
N
(II-1-175)
n'1
where k(n) = 2π/L(n), and L(n) is the wavelength corresponding to the nth frequency f(n); N the total number
of frequency bands of width ∆f. It is not required to divide the spectrum curve equally, except that doing so
greatly facilitates computations. The value of wave height is sensitive to the choice of ∆f, but as long as ∆f
is small, this method produces a satisfactory random wave profile. The use of the equal increments, ∆f,
requires N to be greater than 50 to assure randomness and duplicating the spectrum accurately.
(d) In the non-deterministic spectral amplitude method, the wave surface profile is represented in terms
of two independent Fourier coefficients. These Gaussian distributed random variables an and bn with zero
mean and variance of E(f) ∆f are then obtained from
η(x,t) ' j an cos k (n) x & 2 π f (n) t
N
n'1
(II-1-176)
% j bn sin k (n) x & 2 π f (n) t
N
n'1
(e) In essence, an amplitude and a phase for individual components are replaced by two amplitudes, the
coefficients of cosine and sine terms in the wave profile. This random coefficient scheme may yield a
realistic representation of a Gaussian sea, provided that N is large for a true random sea. This method differs
from the deterministic spectral amplitude approach by ensuring that sea state is Gaussian. Elgar et al. (1985)
have considered simultaneous simulation of both narrow and broad-banded spectra using more than 1000
Fourier components, and concluded that both simulation methods yield similar statistics. These approaches
may be extended to the two-dimensional case. This is beyond the scope of the CEM.
(10) Kinematics and dynamics of irregular waves. In the above sections of the CEM we have considered
definition of irregular wave parameters and development of methods to measure them and use them
analytically. Velocities, pressures, accelerations, and forces under irregular waves are estimated analytically
in three ways. In the first, an individual wave is measured by either a wave-by-wave analysis or constructed
synthetically (such as choosing, Hs, Tz, and a direction) and monochromatic theory is used to estimate the
II-1-98
Water Wave Mechanics


 Previous Page
Previous Page
