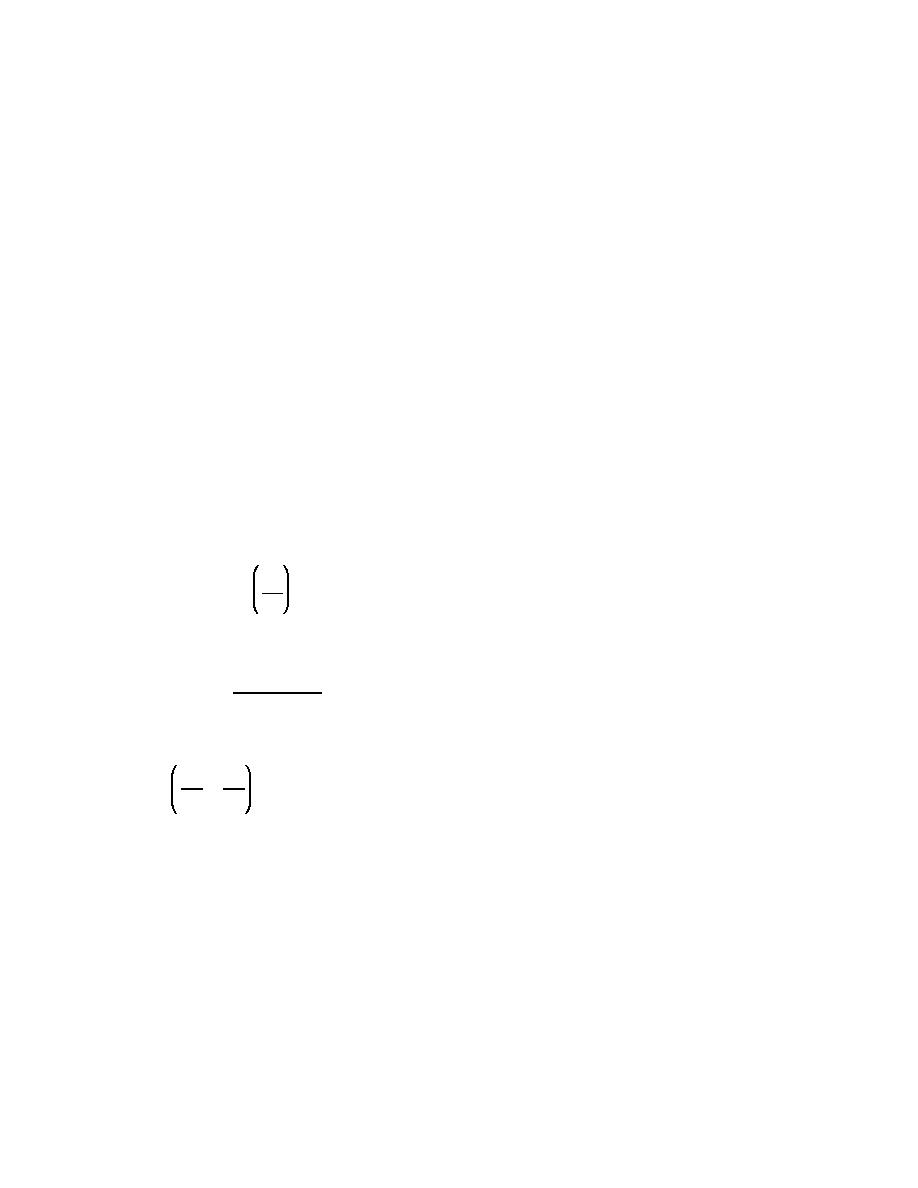
EM 1110-2-1100 (Part II)
30 Apr 02
g. Refraction and shoaling of wave spectra. The previous discussion of refraction and shoaling was for
a single wave component. However, wave propagation was introduced in Equation II-3-1 through the concept
of spectral components. In principle, refraction and shoaling of a wave field in terms of its spectral
components simply requires computing the refraction and shoaling coefficient for each frequency-direction
(f,θ) component and computing the transformed sum:
E (f,θ) ' j Ks (f,θ)
2
2
Kr (f,θ)
Eo (f,θ)
∆f ∆θ
(II-3-23)
where Eo(f,θ) is the offshore directional spectrum. This is possible as long as no breaking or other loss or gain
occurs along the propagation path of the individual waves. If it does occur, most advanced spectral models
compute the wave transformation locally. In this approach, the area of interest is covered by a discrete series
of computation points and the ray path for each (f,θ) component in the spectrum is computed for each grid
point only by tracing the ray back to the grid cell boundary defined by adjacent grid points. This
approximation, called backward ray tracing, is adequate as long as the wave energy and bathymetry vary
smoothly and gently over the domain.
h. Alternate formulations.
(1) Mild slope equation. The refraction and shoaling analyses presented above were based on linear
wave theory and a ray approach equivalent to geometrical optics. This works well for simple cases, but once
the bathymetry becomes even moderately undulatory, the ray approach runs into difficulty. Berkhoff (1972)
formulated a more advanced approach for wave propagation that includes refraction, shoaling, and diffraction
simultaneously and can incorporate structures. Berkhoff developed what is termed the mild-slope equation
given by
Cg
L (CCgΦ) % ω2
Φ'0
(II-3-24)
C
with
cosh k (h % z)
φ (x,y,z) ' Φ
(II-3-25)
cosh kh
where
M
M
L'
(II-3-26)
,
Mxi Myj
which provides a solution φ for amplitude and phase of the waves in the horizontal plane. To obtain the
equation, Berkhoff assumed that the bottom slope was mild (no abrupt steps, shoals, or trenches). Often
slopes of interest violate this assumption, but the models based on the mild-slope equation perform better than
the ray approach. Many approaches have been taken to computationally solve this equation. Berkhoff's
approach solves the velocity potential of the wave in the horizontal, which can require 5-10 computational
grid points per wave length. This is impractical for many cases. Another approach, developed by Radder
(1979), is to use a parabolic approximation, which is far more computationally efficient (but subsequently
adds more limitations).
(2) Boussinesq equations. Another approach for wave propagation problems close to the coast and in
harbors is the use of vertically integrated shallow-water equations in which a Boussinesq (Part II-1)
approximation has been made. The numerical models (e.g., Abbott, Peterson, and Skovgaard 1978) resulting
from this approach require 10-20 grid points per wave length but have the advantage of being time-dependent
Estimation of Nearshore Waves
II-3-17




 Previous Page
Previous Page
