EM 1110-2-1100 (Part II)
30 Apr 02
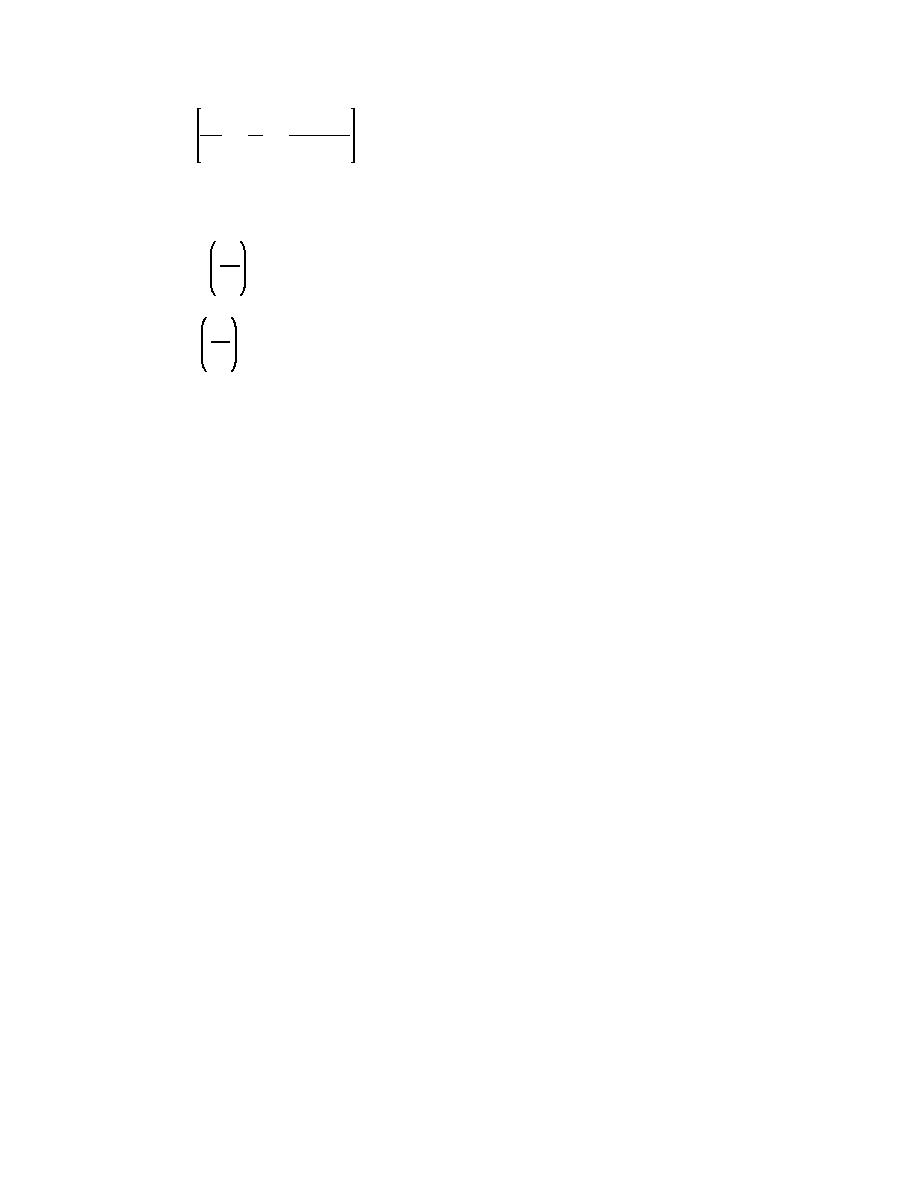
a cos θSX
1
1
VS ' f S
(II-5-13)
&
&
rSX
rS
rS2
where a is the mean radius of the earth. Various geometric relationships are used to write Equations II-5-12
and II-5-13 in the following forms:
a2
VM ' f M
PM
(II-5-14)
3
rM
a2
VS ' f S
PS
(II-5-15)
3
rS
where the terms PM and PS represent harmonic polynomial expansion terms that collectively describe the
relative positions of the earth, moon, and sun. Note that in both cases, the tidal potential term is written as
an inverse function of the distance between the earth and the moon or sun. Both Dronkers (1964) and
Schureman (1924) present detailed derivations of the terms of Equations II-5-14 and II-5-15. For the purpose
of this manual, however, the tidal potential terms shown here are adequate to describe the two most important
features of a tidal record, the spring/neap cycle and the diurnal inequality.
(3) Spring/neap cycle.
(a) The semidiurnal rise and fall of tide can be described as nearly sinusoidal in shape, reaching a peak
value every 12 hr and 25 min. This period represents one-half of the lunar day. Two tides are generally
experienced per lunar day because tides represent a response to the increased gravitational attraction from
the (primarily) moon on one side of the earth, balanced by a centrifugal force on the opposite side of the earth.
These forces create a "bulge" or outward deflection in the water surface on the two opposing sides of the
earth.
(b) The magnitude of tidal deflection is partially a function of the distance between the moon and earth.
When the moon is in perigee, i.e., closest to the earth, the tide range is greater than when it is furthest from
the earth, in apogee. For example, the potential terms in Equation II-5-14 contain the multiplier 1/rM,
describing the distance of the moon from the earth. When the moon is closest to the earth, rM is a minimum
value and the tidal potential term is maximum. Conversely, when the moon is in apogee, the potential term
is at a minimum value. This difference may be as large as 20 percent.
(c) The maximum water surface deflection of semidiurnal tides changes as the relative position of the
moon and sun changes. The amplitude envelope connecting any two successive high tides (and low tides)
gradually increases from some minimum height to a maximum value, and then decreases back to a minimum.
Periods of maximum amplitude are referred to as spring tides, times of minimum amplitude are neap tides.
This envelope of spring to neap occurs twice over a period of approximately 29 days. An example tidal signal
for Boston, MA, is shown in Figure II-5-5 (Harris 1981) in which the normalized tidal signal exhibits two
amplitude envelopes during the total time series.
(d) Spring tides occur when the sun and moon are in alignment. This occurs at either a new moon, when
the sun and moon are on the same side of the earth, or at full moon, when they are on opposite sides of the
earth. Neap tides occur at the intermediate points, the moon's first and third quarters. Figure II-5-6 is a
schematic representation of these predominant tidal phases. Lunar quarters are indicated in the tidal time
II-5-8
Water Levels and Long Waves




 Previous Page
Previous Page
