EM 1110-2-1100 (Part II)
30 Apr 02
advantage for these lower-cost structures is that they may have a relatively high transmission coefficient for
everyday lower waves, but as the height of the incident waves increases, the transmission coefficient will
generally decrease. For harbor installations, they have been used in tandem with a conventional subareal
breakwater placed in their lee - the combined cost of the two structures being less than a single structure with
the same operational criteria (Cox and Clark 1992). A number of laboratory experiments on wave
transmission past submerged rubble-mound structures have been conducted. Results are summarized in
Seelig (1980) and Van der Meer and Angremond (1992).
(b) Van der Meer and Angremond (1992) summarized the available data plus some of their own data to
present a comprehensive procedure for predicting wave transmission for low-crested breakwaters. For
irregular waves they defined the transmission coefficient as the ratio of incident and transmitted significant
breakwaters and negative values for breakwaters with a submerged crest. Correlations with parameters that
also included the incident wave period did not improve results. Figure II-7-19 gives Ct versus F/Hi.
(c) Van der Meer and Angremond (1992) were able to improve the correlations based on experimental
data by introducing the median diameter D50 of the armor stone used to build the structure. (There is a
relationship between D50 and the design wave height for a stable structure.) They then correlated Ct with F/D50
and secondary factors Hs/gTp2, Hi/D50, and B/D50. These relationships are presented in the form of somewhat
complex formulas.
(3) Permeable rubble-mound structures.
(a) Wave energy may transmit through a rubble-mound structure, particularly if it is constructed solely
of a homogeneous mass of large diameter stone. If the structure contains a number of stone layers including
a core of fine stones, the wave transmission will be much less. Also, wave energy transmission through a
stone mound structure would be significant for long-period, low waves, but much less for shorter-period
waves (e.g. the tide would only be slightly reduced by a rubble-mound structure, but steep, wind-generated
waves would have negligible transmission through the structure).
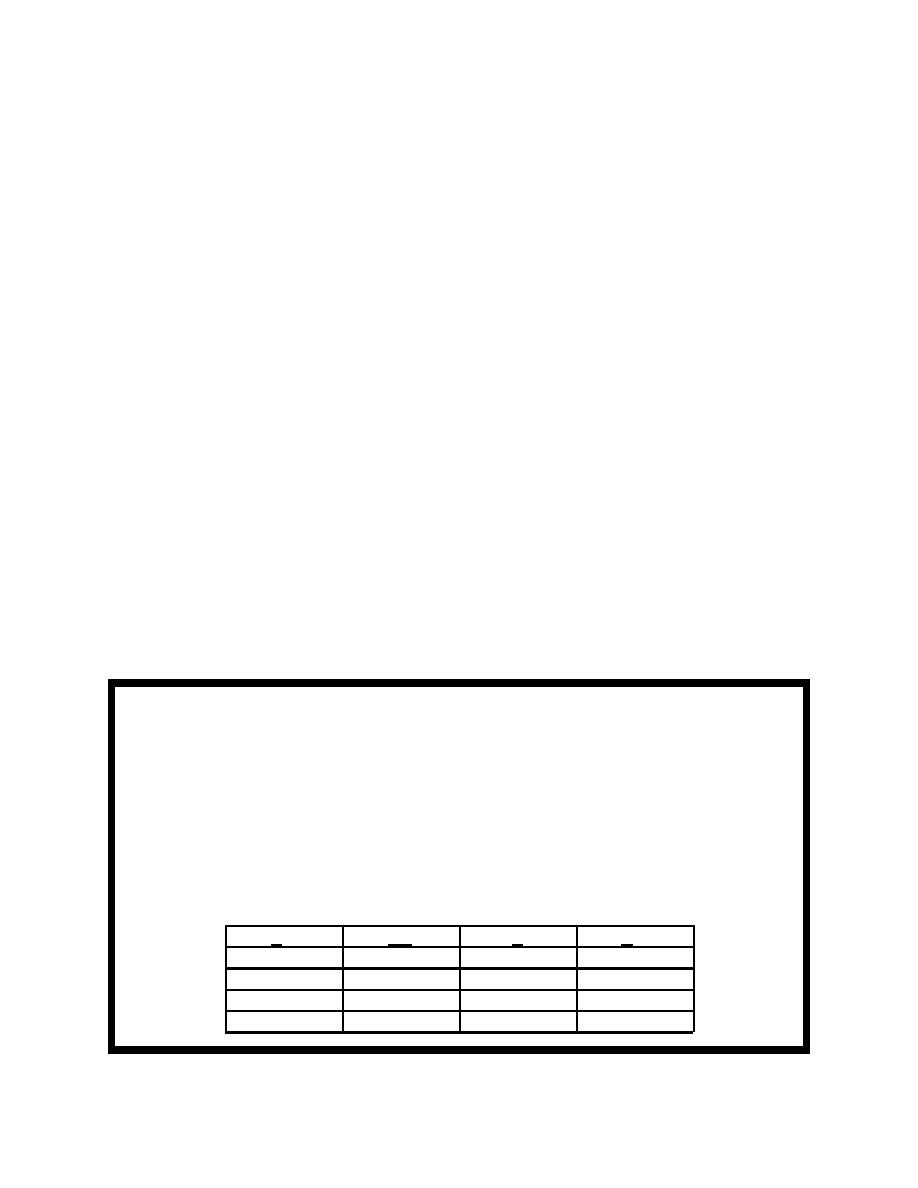
EXAMPLE PROBLEM II-7-2
FIND:
The transmission coefficients and the transmitted wave heights for incident wave heights of 0.5, 1.0, 2.0
and 4.0 m.
GIVEN:
A submerged offshore breakwater situated where the bottom is 4.0 m below the design still-water level.
The structure crest elevation is 3.0 m above the bottom.
SOLUTION:
The breakwater freeboard F is 3.0 - 4.0 = -1.0 m. Employing Figure II-7-19 we have:
F/Hi
Ct
Ht(M)
Hi(m)
0.5
-2.0
0.82
0.41
1.0
-1.0
0.74
0.74
2.0
-0.5
0.60
1.20
4.0
-0.25
0.52
2.10
Harbor Hydrodynamics
II-7-21


 Previous Page
Previous Page
