Wave Reflection

EM 1110-2-1100 (Part II)

30 Apr 02

(b) Wave transmission coefficients for a thin and a relatively wide vertical barrier that has its crest above

or below the water surface were determined from experiments by Goda (1969). The results, which are for

monochromatic waves, are shown in Figure II-7-22. Later tests with irregular waves, where the transmission

coefficient is defined in terms of the incident and transmitted significant wave heights, showed that

Figure II-7-22 can also be used for irregular wave conditions (Goda 2000). As would be expected, a portion

of thetransmitted wave energy shifted to higher frequencies so that the transmitted significant wave period

was less than the incident significant period.

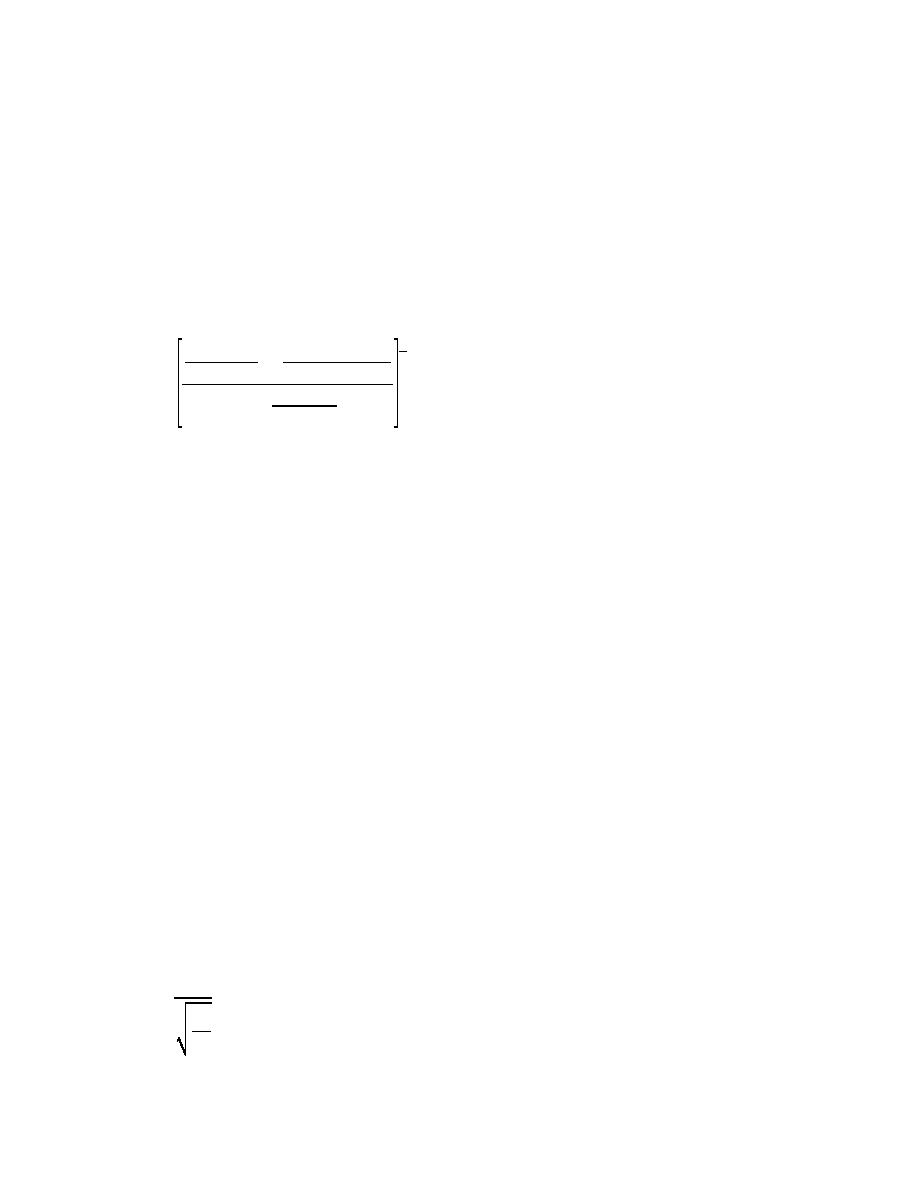
(c) Wiegel (1960) developed a simple analytical formulation for wave transmission past a thin rigid

vertical barrier that does not extend to the bottom. For monochromatic waves and no overtopping, the

resulting transmission coefficient is given by

1

2 k (d & y)

sinh 2 k (d & y)

2

%

sinh 2 k d

Ct '

(II-7-5)

2 kd

1%

sinh 2 k d

where d is the water depth, y is the vertical extent of the barrier below the still-water surface, and k = 2π/L.

In developing Equation II-7-5, Wiegel assumed that the portion of the wave power in the water column below

the lower edge of the barrier transmits past the barrier. He used the linear wave theory in this analysis and

neglected energy dissipation caused by flow separation at the barrier edge. Limited monochromatic wave

tests by Wiegel (1960) and irregular wave tests by Gilman and Nottingham (1992) indicate that Equation II-7-

5 can be used for preliminary design calculations.

II-7-4. Wave Reflection

a. Definition of reflection.

(1) If there is a change in water depth as a wave propagates forward, a portion of the wave's energy will

be reflected. When a wave hits a vertical, impermeable, rigid surface-piercing wall, essentially all of the wave

energy will reflect from the wall. On the other hand, when a wave propagates over a small bottom slope, only

a very small portion of the energy will be reflected. The degree of wave reflection is defined by the reflection

coefficient Cr = Hr/Hi where Hr and Hi are the reflected and incident wave heights, respectively.

(2) Wave energy that enters a harbor must eventually be dissipated. This dissipation primarily occurs

at the harbor interior boundaries. Thus, it is necessary to know the reflection coefficients of the interior

boundaries to fully define wave conditions inside a harbor. It may also be necessary, because of excessive

wave reflection, to decrease the reflection of certain boundary structures in order to keep interior wave

agitation at acceptable levels.

(3) The reflection coefficient for a surface-piercing sloped plane will depend on the slope angle, surface

roughness, and porosity. It will also depend on the incident wave steepness Hi/L. Consequently, for a given

slope roughness and porosity, the wave reflection will depend on a parameter known as the surf similarity

number or Iribarren number (Battjes 1974)

tanα

Ir '

(II-7-6)

Hi

Lo

II-7-26

Harbor Hydrodynamics