Wave energy and power.

EM 1110-2-1100 (Part II)

30 Apr 02

4πd

1

L

n'

1%

(II-1-50)

2

4πd

sinh

L

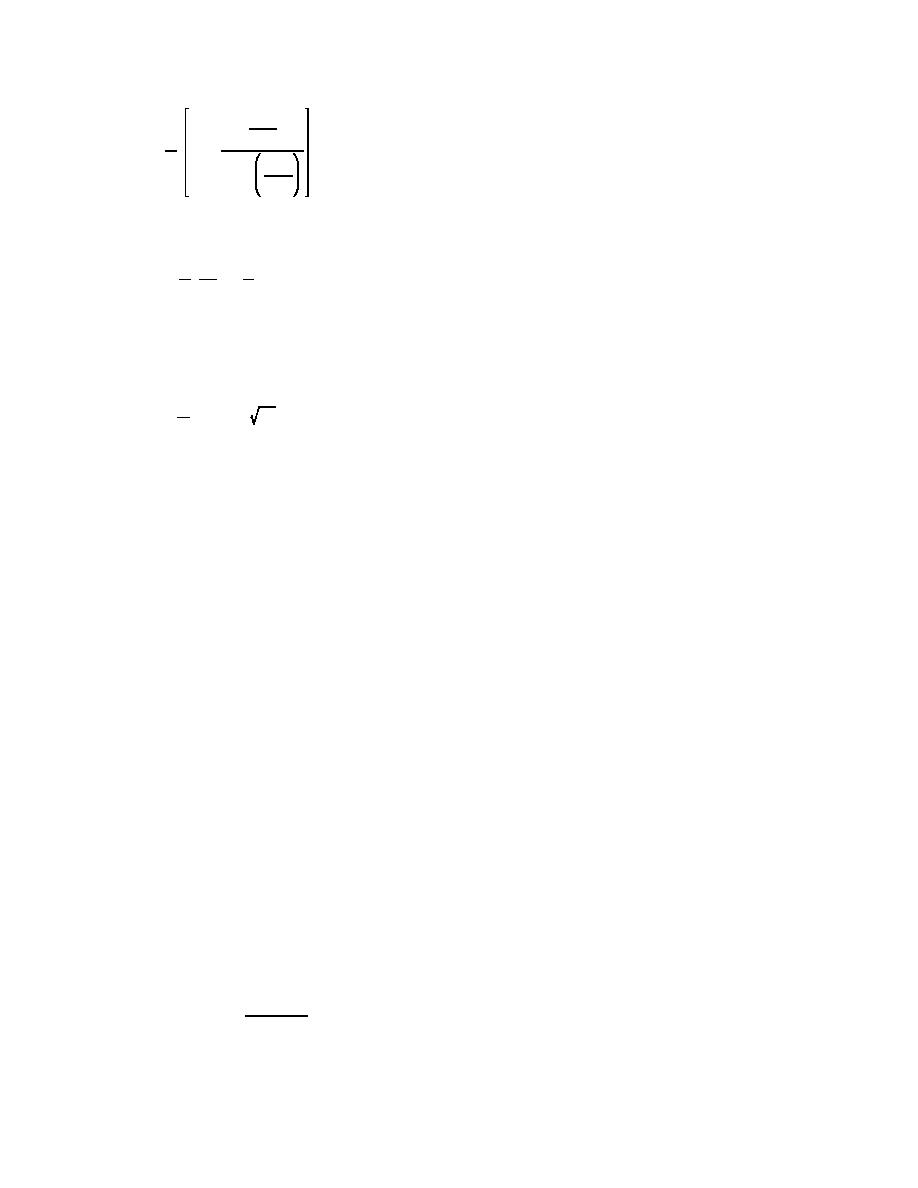
(d) In deep water, the term (4πd/L)/sinh(4πd/L) is approximately zero and n = 1/2, giving

1 L0

1

C0 (deep water)

Cg '

(II-1-51)

'

2 T

2

0

or the group velocity is one-half the phase velocity.

(e) In shallow water, sinh(4πd/L . 4πd/L) and

L

' C . gd (shallow water)

Cg '

(II-1-52)

T

s

hence, the group and phase velocities are equal. Thus, in shallow water, because wave celerity is determined

by the depth, all component waves in a wave train will travel at the same speed precluding the alternate

reinforcing and canceling of components. In deep and transitional water, wave celerity depends on

wavelength; hence, slightly longer waves travel slightly faster and produce the small phase differences

resulting in wave groups. These waves are said to be dispersive or propagating in a dispersive medium; i.e.,

in a medium where their celerity is dependent on wavelength.

(f) The variation of the ratios of group and phase velocities to the deepwater phase velocity Cg/C0 and

C/C0, respectively are given as a function of the depth relative to the deep water wavelength d/L0 in

Figure II-1-7. The two curves merge together for small values of depth and Cg reaches a maximum before

tending asymptotically toward C/2.

(g) Outside of shallow water, the phase velocity of gravity waves is greater than the group velocity. An

observer that follows a group of waves at group velocity will see waves that originate at the rear of the group

move forward through the group traveling at the phase velocity and disappear at the front of the wave group.

(h) Group velocity is important because it is with this velocity that wave energy is propagated. Although

mathematically the group velocity can be shown rigorously from the interference of two or more waves

(Lamb 1945), the physical significance is not as obvious as it is in the method based on the consideration of

wave energy. Therefore an additional explanation of group velocity is provided on wave energy and energy

transmission.

(9) Wave energy and power.

(a) The total energy of a wave system is the sum of its kinetic energy and its potential energy. The

kinetic energy is that part of the total energy due to water particle velocities associated with wave motion.

The kinetic energy per unit length of wave crest for a wave defined with the linear theory can be found from

u2 % w2

m&d

mx

x%L

η

ρ

dz dx

Ek '

(II-1-53)

2

II-1-26

Water Wave Mechanics