EM 1110-2-1100 (Part II)
30 Apr 02
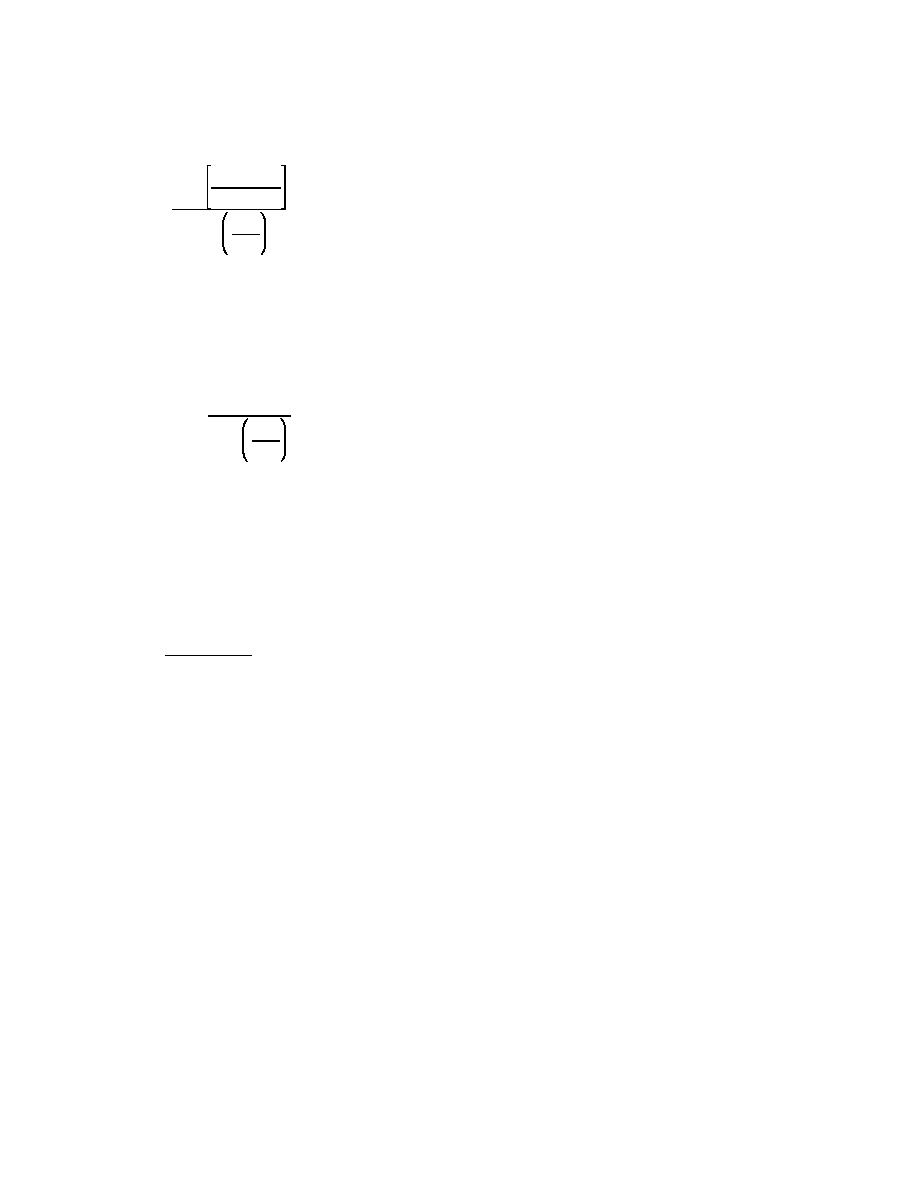
(c) The ratio
2π(z % d)
cosh
L
Kz '
(II-1-43)
2πd
cosh
L
is termed the pressure response factor. Hence, Equation II-1-41 can be written as
p ' ρg(ηKz & z)
(II-1-44)
(d) The pressure response factor K for the pressure at the bottom when z = -d,
1
Kz ' K '
(II-1-45)
2πd
cosh
L
is presented as function of d/L0 in the tables (SPM 1984); see also Figure II-1-5. This figure is a convenient
graphic means to determine intermediate and shallow-water values of the bottom pressure response factor K,
the ratio C/C0 (=L/L0 = k0 /k ), and a number of other variables commonly occurring in water wave
calculations.
(e) It is often necessary to determine the height of surface waves based on subsurface measurements of
pressure. For this purpose, it is convenient to rewrite Equation II-1-44 as
N (p % ρ g z)
η'
(II-1-46)
ρ g Kz
where z is the depth below the SWL of the pressure gauge, and N a correction factor equal to unity if the
linear theory applies.
(f) Chakrabarti (1987) presents measurements that correlate measured dynamic pressure in the water
column (s in his notation is the elevation above the seabed) with linear wave theory. These laboratory
measurements include a number of water depths, wave periods, and wave heights. The best agreement
between the theory and these measurements occurs in deep water. Shallow-water pressure measurements for
steep water waves deviate significantly from the linear wave theory predictions. The example problem
hereafter illustrates the use of pertinent equations for finding wave heights from pressure measurements based
on linear theory.
(8) Group velocity.
(a) It is desirable to know how fast wave energy is moving. One way to determine this is to look at the
speed of wave groups that represents propagation of wave energy in space and time. The speed a group of
II-1-22
Water Wave Mechanics


 Previous Page
Previous Page
