EM 1110-2-1100 (Part II)
30 Apr 02
16d 3
L'
(II-1-80)
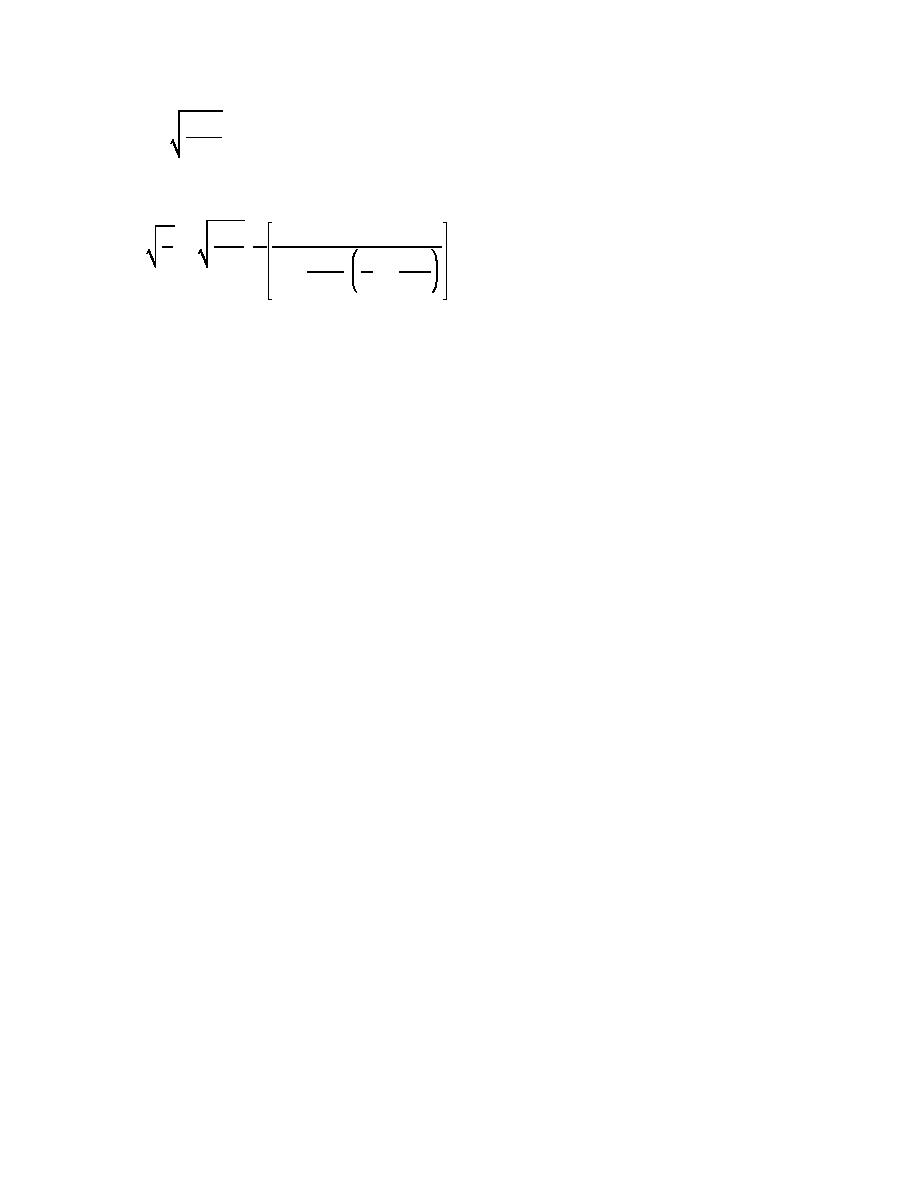
k K(k)
3H
and wave period by
16yt d
g
k K(k)
T
(II-1-81)
'
3H yt
d
H
1
E(k)
1%
&
2
K(k)
yt k 2
Note that cnoidal waves are periodic and of permanent form; thus L = CT (see Figure II-1-10).
(j) Pressure under a cnoidal wave at any elevation y above the bottom depends on the local fluid
velocity, and is therefore complex. However, it may be approximated in a hydrostatic form as
p ' ρg (ys & y)
(II-1-82)
i.e., the pressure distribution may be assumed to vary linearly from ρgys at the bed to zero at the surface.
(k) Wave profiles obtained from different wave theories are sketched in Figure II-1-10 for comparison.
The linear profile is symmetric about the SWL. The Stokes wave has higher more peaked crests and shorter,
flatter troughs. The cnoidal wave crests are higher above the SWL than the troughs are below the SWL.
Cnoidal troughs are longer and flatter and crests are sharper and steeper than Stokes waves. The solitary
wave, a form of the cnoidal wave described in the next section, has all of its profile above the SWL.
(l) Figures II-1-11 and II-1-12 show the dimensionless cnoidal wave surface profiles for various values
of the square of the modulus of the elliptic integrals k2, while Figures II-1-13 to II-1-16 present dimensionless
plots of the parameters which characterize cnoidal waves. The ordinates of Figures II-1-13 and II-1-14 should
be read with care, since values of k2 are extremely close to 1.0 (k2 = 1 - 10-1 = 1 - 0.1 = 0.90). It is the
exponent α of k2 = 1 - 10-α that varies along the vertical axis of Figures II-1-13 and II-1-14.
(m) Ideally, shoaling computations might be performed using a higher-order cnoidal wave theory since
this theory is able to describe wave motion in relatively shallow water. Simple, completely satisfactory
procedures for applying cnoidal wave theory are not available. Although linear wave theory is often used,
cnoidal theory may be applied for practical situations using Figures such as II-1-11 to II-1-16. The following
problem illustrates the use of these figures.
(n) There are two limits to the cnoidal wave theory. The first occurs when the period of the function
cn is infinite when k = 1. This corresponds to a solitary wave. As the wavelength becomes infinite, the
cnoidal theory approaches the solitary wave theory. The second limit occurs for k = 0 where the cnoidal
wave approaches the sinusoidal wave. This happens when the wave height is small compared to water depth
and the cnoidal theory reduces to the linear theory.
Water Wave Mechanics
II-1-39


 Previous Page
Previous Page
