EM 1110-2-1100 (Part II)
30 Apr 02
reflects completely from the structure. Wiegel (1962) summarizes the Penny and Price (1952) solution and
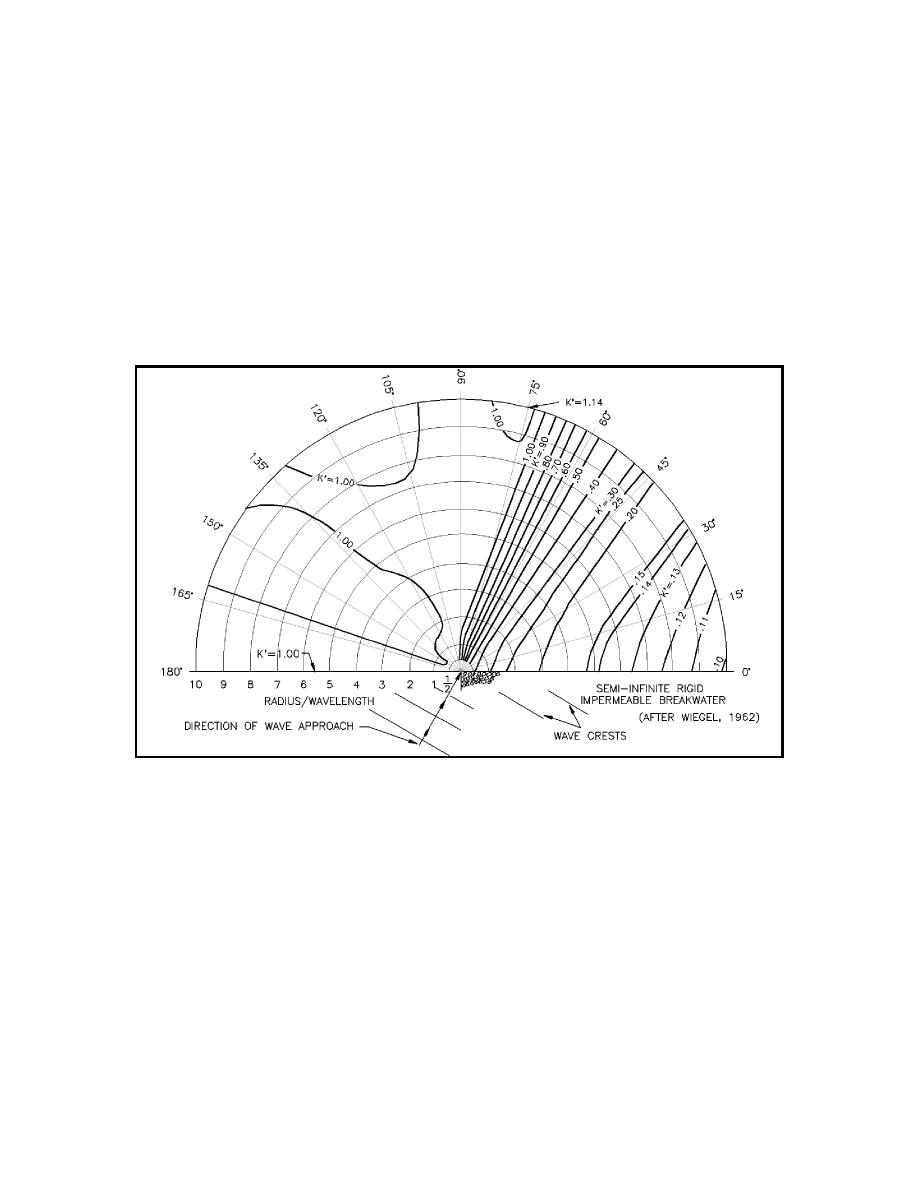
tabulates results of this solution (KN = fct(r/L, β, θ) for selected values of r/L, β and θ. Figure II-7-3 shows
Wiegel's (1962) results for an approach angle θ of 60 deg. Plots of approach angles θ varying by 15-deg
intervals from 15 to 180 deg can be found in Wiegel (1962) and the Shore Protection Manual (1984).
(e) An interesting feature demonstrated by Figure II-7-3 is that for this approach angle, the value of the
diffraction coefficient along a line in the lee of the breakwater that extends from the breakwater tip in the
direction of the approaching wave is approximately 0.5. This is true not only for the approach angle of
60 deg, but for any approach angle. Note also that for a given location in the lee of a breakwater, a one-
dimensional spectrum of waves that comes from the same direction will undergo a greater decrease in
height(energy density) for successively higher frequency waves in the spectrum. Increasing frequencies mean
shorter wavelengths and consequently larger values of r/L (for given values of β and θ). Thus the diffracted
spectrum will have a shift in energy density towards the lower frequency portion of the spectrum.
Wave diffraction diagram - 600 wave angle
Figure II-7-3.
(f) Wave tank measurements of diffracted heights for waves passing a semi-infinite barrier were made
by Putnam and Arthur (1948). They considered six approach directions for each of two incident wave
periods. Their measurements generally confirm the diffraction theory. But, the diffraction theory assumes
small-amplitude waves and Putnam and Arthur (1948) employed relatively small-amplitude waves in their
experiments. For steeper waves, finite amplitude effects would cause the results to differ somewhat from the
diffraction theory based on small-amplitude waves.
Harbor Hydrodynamics
II-7-5


 Previous Page
Previous Page
