EM 1110-2-1100 (Part II)
30 Apr 02
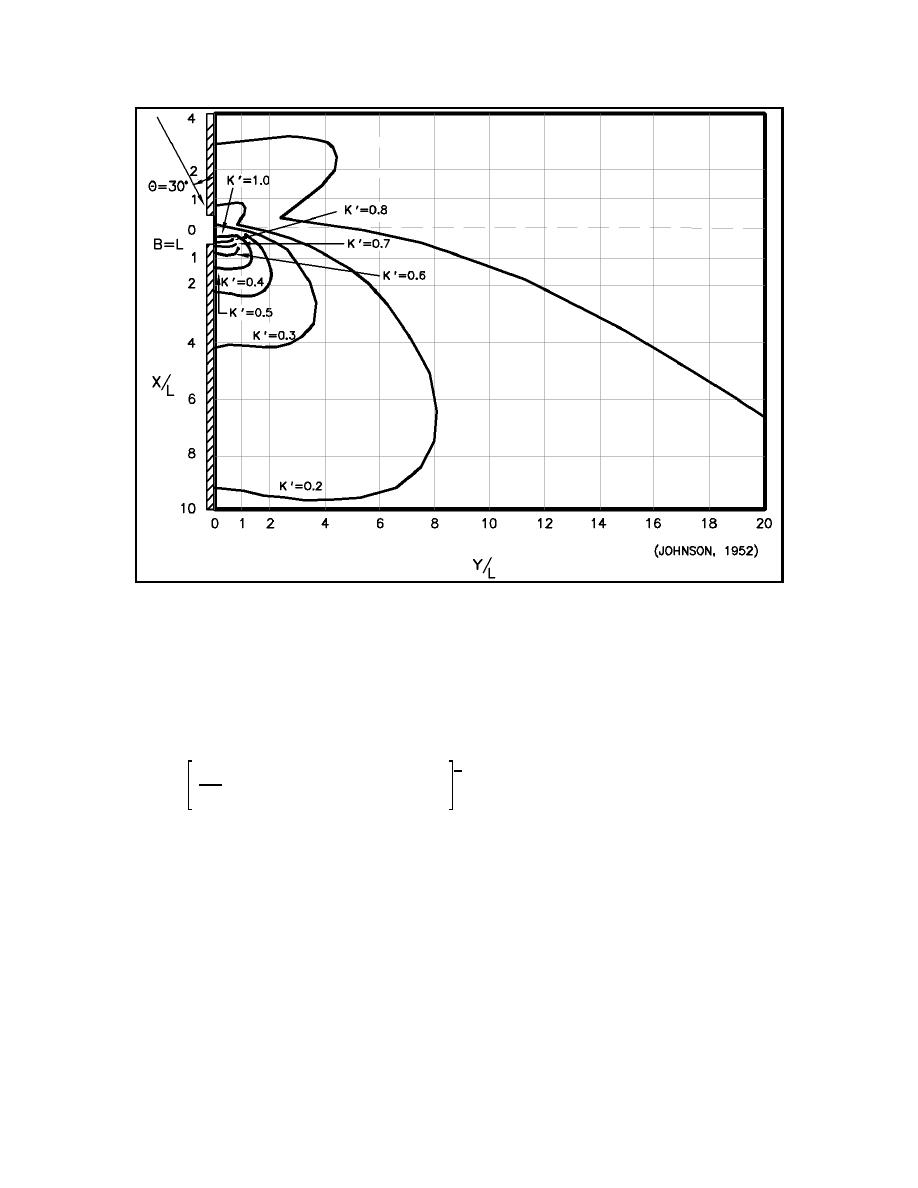
Figure II-7-9.
Diffraction for a breakwater gap of one wavelength width where φ = 30 deg
(2) To evaluate the effect of diffraction on a directional wave spectrum, Goda, Takayama, and Suzuki
(1978) calculated diffraction coefficients for a semi-infinite breakwater and a breakwater gap by breaking the
spectrum into number of frequency (10) and direction (20 to 36) components and combining the result at
points in the breakwater lee. This produced an effective diffraction coefficient defined by
1
1
Mo m0 mθ min
4
θ max
)
S(f,θ) (K ) )2 d θ df
2
Ke
(II-7-1)
'
where KN is the diffraction coefficient for each frequency/direction component when acting as a
monochromatic wave, M0 is the zero moment of the spectrum, df and dθ are the frequency and direction
ranges represented by each component of the spectrum, θmax and θmin are the limits of the spectral wave
component directions, and S(f,θ) is the spectral energy density for the individual components. The spectral
frequency distribution they employed was similar to most typical storm spectra such as the JONSWAP
spectrum. The directional spread of the spectrum was characterized by a directional concentration parameter
Smax, which equals 10 for widely spread wind waves and 75 for swell with a long decay distance, so the
directional spread is quite limited.
II-7-10
Harbor Hydrodynamics


 Previous Page
Previous Page
