EM 1110-2-1100 (Part II)
30 Apr 02
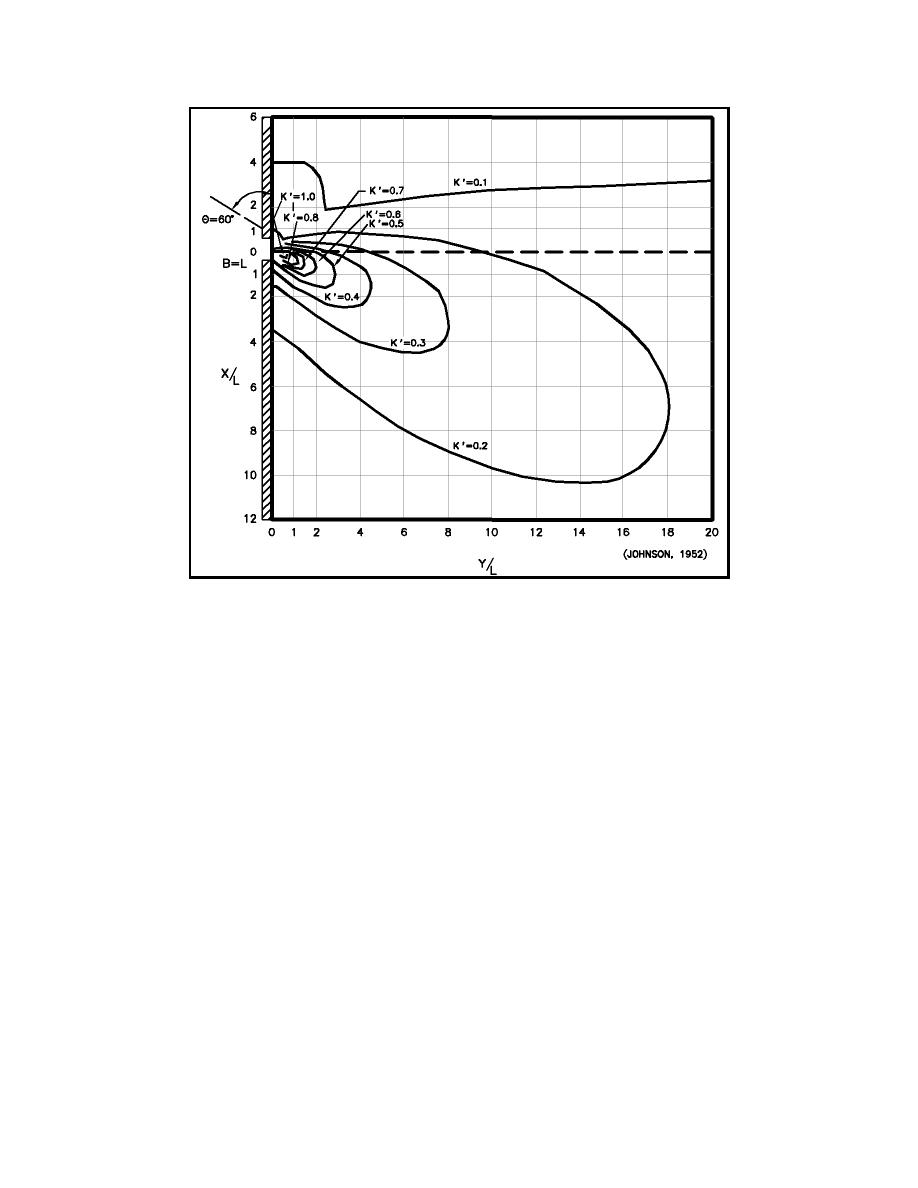
Figure II-7-11. Diffraction for a breakwater gap of one wavelength width where
φ = 60 deg
e. Combined refraction-diffraction in harbors.
(1) In most harbors, the depth is relatively constant so the diffraction analyses discussed above are
adequate to define the resulting wave conditions. However, if the depth changes significantly, then wave
amplitudes will change because of shoaling effects. If the harbor bottom contours are not essentially parallel
to the diffracting wave crests, then wave amplitudes and crest orientations will be affected by refraction.
(2) Where depth changes in a harbor are sufficient for combined refraction and diffraction effects to be
significant, the resulting wave height and direction changes can be investigated by either a numerical or a
physical model study. For examples of numerical model studies of combined refraction-diffraction in the lee
of a structure, see Liu and Lozano (1979), Lozano and Liu (1980), and Liu (1982). Physical models that
investigate the combined effects of refraction and diffraction are routinely conducted (see Hudson et al.
1979). The one major limitation on these models for wind wave conditions is that the model cannot have a
distorted scale (i.e., horizontal and vertical scale ratios must be the same). Sometimes, lateral space
limitations or the need to maintain an adequate model depth to avoid viscous and surface tension scale effects
make a distorted scale model desirable. But such a model cannot effectively investigate combined refraction-
diffraction problems.
(3) In many cases, the depth near the entrance to a harbor is relatively constant with the significant
depth changes occurring further from the entrance (in the vicinity of the shoreline). Then an approximate (but
often adequate) analysis can be carried out using the techniques discussed herein. The diffraction analysis
would be carried out from near the harbor entrance to the point inside the harbor where significant
II-7-12
Harbor Hydrodynamics


 Previous Page
Previous Page
